Complementary and Supplementary Angles Worksheets
We now begin to work on the foundational skills for writing geometric proofs. This basic vocabulary and being able to identify angle relationships such as these will help you form logical arguments to understand geometric shapes and finding unknown lengths of sides and measures within the context of a geometry-based situation. You can use this skill to determine the angle of a cut or length a decking board or flooring tile should be when you are working on your home. All types of construction rely on this form of geometry to make structures sturdy and level. Through the use of these worksheets and lessons students learn how to identify these types of angle relationships as well as use them to their advantage to decipher the value of other missing or unknown angles.
Aligned Standard: 7.G.B.5
- Complementary and Supplementary Angles Lesson- The problems are not visual, but we explain the answers visually to help out.
- Find That X Guided Lesson - We present each problem in the form of a graphic.
- Guided Lesson Explanation - Yeah, you need a little bit of algebra to get this working for you.
- Find and Define the Angles Worksheet 1 - We really give them a what for here. There is a really good mix of skills explored here.
- Sup! Angles Worksheet 2 - The algebra involved are all two-step problems.
- Mixed Review 4-Pack - You will find visual angles, have some time with matching and also just do some math too.
- Answer Keys - These are for all the unlocked materials above.
Homework Sheets
The homework is a building series and really enhances their skills.
- Homework 1 - Angles are referred to as supplementary when they add up to 180°.
- Homework 2 - Are the angles supplementary, complementary or neither? Use "S", "C", and "N" to show the angle relationship.
- Homework 3 - Find the value of x for each linear pair of angles. You can use your knowledge of their relationship to help you through.
Practice Worksheets
Not many people realize how critical this skill is in the real world. Thousands of buildings are standing because of it.
- Practice 1 - Angles are referred to as complementary when they add up to 90°.
- Practice 2 - Find the complement to each of the angles. Which one might that be?
- Practice 3 - If we have that 34°, the complementary angle would be defined as x in the equation 34° + x = 90°
Math Skill Quizzes
When we built my backyard deck, we used this skill dozens of times a day. Math the unsung hero!
- Quiz 1 - If we have that 67°, the supplementary angle would be defined as x in the equation 67° + x = 180°
- Quiz 2 - We tell that the missing angle is formed when a diagonal line passes across a perfectly straight horizontal line (given).
- Quiz 3 - Complete the chart with the complementary and supplementary angles to each given angle. If there is no complement or supplement is possible state "None".
What Are Complementary and Supplementary Angles?
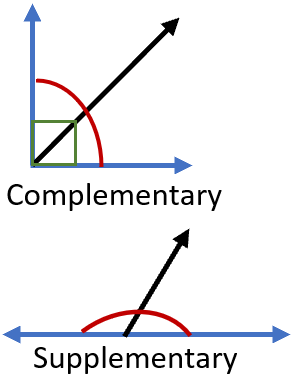
An angle is a way of defining the relationship between two lines that start at the same point. In geometry, an angle is described as a figure formed by two rays meeting at the vertex. An angle is represented by the symbol ∠.
Several types of relationships that can be formed by using the angles. Below, we have discussed the most common type of relationship between two angles.
Complementary Angle - Complementary angles are formed by two angles that add up to 90 degrees. When studying the complementary angles, it is crucial to remember that they come in pairs. While talking about one of the angles in complementary pair, we say that one angle is complementary of the other or is the complement of the other. Complementary angles always have a positive measure. Since these angles add up to 90 degrees, the two angles forming a complementary angle area always acute. As you advance on with geometry this will become a foundational term that will help you when crafting geometric proofs. If you see a right angle available, when writing proofs, you can pretty much bet the farm this this term will be included.
Supplementary Angle - Supplementary angles are formed when two angles add up to 180 degrees. The two angles forming a supplementary angle make a straight angle. When the sum of two angles is 180 degrees, we say that they are a supplement to each other. Once again this is an essential for your more advanced proofs.
The Importance of Describing Angle Relationships
Being able to recognize connections between different angles has a great deal value for you as you approach geometry and other practical applications of math. You can use this skill to tell you the measures of angles you do not know without ever measuring a thing. While this a great skill to have to do well on test, it also has huge real-world implications for you. Whether you realize it or not people that work on construction jobs use these concepts every single day. Imagine building a deck and calculating how to make a perfect curve or bend around a house or obstacle. This is where understanding angle relationships is key, it will allow you to understand some many other measures with little to no effort. This math skill also has application in laying tile, installing sheet rock, you would be hard pressed to find situations where it does not have application to wood working when building or fixing up a home.